What Is A Common Tangent

The common-tangent problem is named for the single tangent segment that's tangent to two circles. Your goal is to observe the length of the tangent. These bug are a bit involved, but they should cause y'all fiddling difficulty if you utilise the straightforward iii-step solution method that follows.

The following example involves a common external tangent (where the tangent lies on the same side of both circles). Y'all might also see a common-tangent problem that involves a common internal tangent (where the tangent lies between the circles). No worries: The solution technique is the aforementioned for both.

Here's how to solve it:

Draw the segment connecting the centers of the two circles and draw the ii radii to the points of tangency (if these segments haven't already been drawn for y'all).

The post-obit effigy shows this pace. Note that the given altitude of eight between the circles is the distance betwixt the outsides of the circles along the segment that connects their centers.
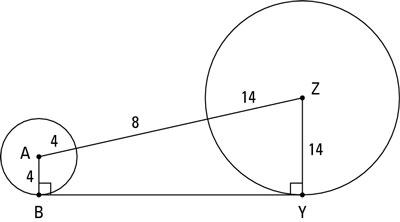
From the centre of the smaller circumvolve, depict a segment parallel to the common tangent till it hits the radius of the larger circle (or the extension of the radius in a common-internal-tangent problem).

You end up with a right triangle and a rectangle; i of the rectangle's sides is the common tangent. The following figure illustrates this step.
You now have a right triangle and a rectangle and can finish the problem with the Pythagorean Theorem and the elementary fact that reverse sides of a rectangle are coinciding.

The triangle's hypotenuse is fabricated upwards of the radius of circle A, the segment between the circles, and the radius of circumvolve Z. Their lengths add up to four + eight + 14 = 26. Y'all tin can come across that the width of the rectangle equals the radius of circle A, which is 4; because opposite sides of a rectangle are coinciding, you can then tell that one of the triangle's legs is the radius of circumvolve Z minus iv, or 14 – 4 = 10. You at present know two sides of the triangle, and if you notice the tertiary side, that'll give you the length of the common tangent. You go the third side with the Pythagorean Theorem:

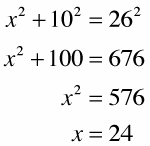
(Of course, if you recognize that the right triangle is in the 5 : 12 : 13 family, you can multiply 12 past 2 to get 24 instead of using the Pythagorean Theorem.)

Because opposite sides of a rectangle are congruent, By is also 24, and yous're done.

Now wait back at the last figure and note where the correct angles are and how the right triangle and the rectangle are situated; and so make sure you mind the post-obit tip and alert.

Annotation the location of the hypotenuse. In a common-tangent problem, the segment connecting the centers of the circles is ever the hypotenuse of a correct triangle. The common tangent is always the side of a rectangle, not a hypotenuse.

In a common-tangent problem, the segment connecting the centers of the circles is never ane side of a right angle. Don't make this mutual mistake.